The purpose of this experiment was to gain knowledge and understanding of sound waves by examing their characteristics such as the number of wave, frequency, period, amplitude, and wavelength. The relationships between these variables were calculated through different sound waves.
Part 1
Set up
First person said "AAAAAA"
Wave #1
Questions answered
Wave #1h (sample was 10 times longer than #1)
Graph #1 showed that the prediction was consistent with the experiment.
Part 2
Someone else said "AAAAA"
Wave # 2
Questions answered
Part 3
Tunning fork wave collected
Wave # 3
The major difference of waves between human voice and a tunning fork: human voice wave is not sinusoidal (superposition of different waves) but a tunning fork wave is sinusoidal.
Part 4
Wave # 4: tunning fork created a soft sound.
Wave 3 and 4 were created by a sound from the same tunning fork but with different loudness; therefore, they were expected to have the same wavelength and frequency but different amplitude because the loudness is dependent on the amplitude of the wave. The sound of tunning fork was made softer by striking it softer. The experimental data proved that the louder sound has a larger amplitude.
















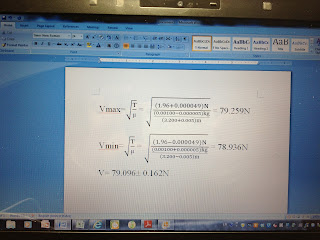






.png)







